Quadratics
Grade 10 Math
Shiyana Veerasingam
Standard Form is written as y= ax2+bx+c and can be factored, changed to vertex form and graphed as a quadratic function.
Standard Form
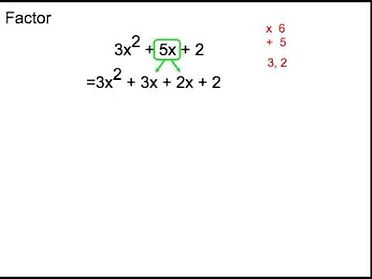
CONVERTING STANDARD TO FACTORED FORM
Standard Form to Vertex Form
To convert standard form to vertex form their should be a perfect square, if not create your own.
If the given equation already has a perfect square, the only thing that needs to be done is factoring. When factored, youll get the vertex and the next step would be to plug it into the vertex form y=a(x-h)+k. The x value of the vertex would be where h is and the y value of the vertex would be where k is.
For instance y=x2+2x+1
y=(x+1)(x+1)
The vertex would be (-1,0)
When a given equation is not a perfect square, create your own. For exapmle, if an equation like y=x2+2x is given add in your own by dividing bx by 2 then squaring then cancel out the same number from the equation so the whole equation itself doesnt get affceted. So back to our example (2x/2)2 is 1 so theirfor the entire equation would look like y=x2+2x+1-1. The next step would be to factor, but make sure you ignore the -1. When factored bring back the -1 and then the equation would look like y=(x+1)2 -1. Now the vertex is given which is (-1,-1) plug it into vertex form.
Another case of converting standard form to vertex form would be if an equation is given and c cannot be squared nor do its factor equal bx. An example would be 3x2+6x+5 to solve this equations there is a step by step procedure below:
Step one: Move c out of the picture
y=3x2+6x
Step two: Factor out the first coefficient
y=3(x2+2x)
Step three: Completing the Square, (b/2)2 would be the replacement for c
(2x/2)2=1
y=3(x2+2x+1)
Step four: Cancel out the number you just inputted into the equation by expanding the 3 into the bracket
y=3(x2+2x+1)-3
Step five: Factor the equation
y=3(x+1)(x+1) -3
Step six: Bring back the c
y=3(x+1)2 -3+5
Step seven: collect like terms
y=3(x+1)2 +2
So now the vertex would be (-1,+2)
Example of Simple Trinomial Factoring:
Equation: x2+7x+6
1) Determine values for ax2, bx and c from the equation provided.
ax2=x2
bx=7x
c=6
2) Find out all the posible factors of c.
1x6=6
2x3=6
3x2=6
6x1=1
3) Next, go through all the possibities listed above and check if either of them add up to bx.
1+6=7
2+3=5
3+2=5
6+1=7
4) Find out what two coefficients mutilply to equal ax2.
x*x=x2
5) Now that the two numbers multiply to equal c and add up to equal bx and we know that the coefficients mutiply to ax2, we need to re-write our equation into two binomials.
(x+1)(x+6)
Example of Complex Trinomial Factoring:
The difference between simple and complex factoring is that in simple trinomials ax2 is equal to 1. If ax2 happens to be greater than 1, it is complex factoring.
There is not much of a differnce between factoring a simple trinomial and a complex trinomial. Follow the first three steps listed above for simple trinomials for complex factoring. The only step that changes is step four. Please refer below.
1)-3) Follow the same steps as outlines in simple trinomial factoring.
4) Find out what two coefficients mutiply to equal ax2.
Since there maybe a variety of pairs, you need to plug in all of your factors which mutiply to c and add up to bx into your equation with your coefficients.
CHECK YOUR ANSWER
To check if your answer is correct, expand your brackets. When the expansion is complete, the equation should be the same as the standard equation you started with.
(x+1)(x+6)
=x2+6x+1x+6
Now you collect like term
=x2+7x+6